To find the position of 1002, we must delete all multiples of 7, all multiples of 11 and all multiples of 13 from 1 to 1002. We apply principle of inclusion and exclusion to find that:

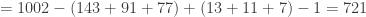
Hence the position of 1002 is 721 in the list.
To find the number in the 3600 position, we assume that it is x. Then

Note that 7*11*13 = 1001 Hence from 1 to 1001 there are exactly 720 numbers (as found in Part 1) which are not divisible by 7, 11, 13. Similarly in the next 1001 numbers (from 1002 to 2002) we will have another 720 numbers which are not divisible by 7, 11, 13. To reach 3600 we have to do this exactly 5 times as 720 * 5 = 3600 . Hence there are 3600 numbers from 1 to 1001*5 = 5005.
Thus the 3600th number is 5004.